You Can’t Resist This: Exploring Resistance within Electronic Systems

Ever wonder what makes electronics tick? What goes inside circuits and systems that make everything work? Every electronic system consists of three major elements: voltage, current, and resistance. In this blog, we will explore resistance, what it is, its history, and how to use it.

In this Blog,

1. History

2. Resistor

3. Resistor in Series

4. Resistor in Parallel

5. Voltage Divider

6. Current Divider

7. Measure Voltage and Current

8. Conclusion

History

Electrical resistance was first discovered and formulated by German physicist Georg Simon Ohm in 1827. Ohm's groundbreaking work, Ohm's Law, laid the foundation for understanding the relationship between voltage, current, and resistance in electrical circuits. His invention of the resistor, a device specifically designed to provide a controlled amount of resistance in an electrical circuit, revolutionized the field of electrical engineering.

Resistor

A resistor is simply a passive two-terminal element that reduces current flow and divides voltages. Imagine a water pipe where the flow of water is equal to an electrical current. The flow is reduced when the diameter of the pipe is reduced, adding resistance into the system.

Resistor in Series

A series of resistors is when a resistor is connected from one terminal to another in succession in an electrical circuit. This arrangement is known as resistors in series, as shown in Figure 1. In this configuration, the total resistance is the sum of the individual resistances (Riedel & Nilsson, 2015, 58).

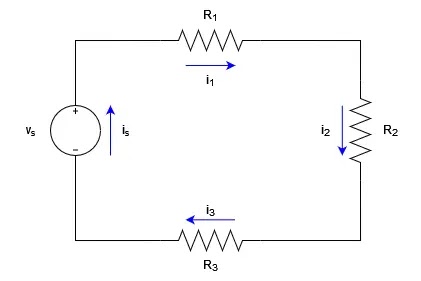Figure 1

Due to the nature of series circuit, the current in a closed loop equals the current source (i_s )

i_s = i₁ = i₂ = i₃ (1)

By applying Kirchhoff's voltage law (KVL), the voltage (v_s)can be obtained by,

-v_s + i_sR₁ + i_sR₂ + i_sR₃ = 0 (2)

v_s = i_s(R₁ + R₂ + R₃) (3)

Therefore, the equivalent resistance is,

R_eq = R₁ + R₂ + R₃ (4)

Resistor in Parallel

Contrary to resistors in series, resistors in parallel are connected with both terminals side by side in a circuit. In this configuration, the total resistance is calculated differently. The reciprocal of the total resistance is equal to the sum of the reciprocals of the individual resistances (Riedel & Nilsson, 2015, 59). Parallel resistors allow current to divide among multiple paths, providing flexibility and load-sharing capabilities in electrical systems.

Figure 2

Due to the nature parallel circuit, the voltage across each resistor equals the source voltage (v_s)

v_s = v_R1 = v_R2 = v_R3 (5)

i_R1R₁ = i_R2R₂ = i_R3R₃ = v_s (6)

iR₁ = v_s/R₁; iR₂ = v_s/R₂; iR₃ = v_s/R₃ (7)

By applying Kirchhoff’s current law (KCL), the current (i_s) can be obtained by,

i_s  = iR₁ + iR₂ + iR₃ (8)

Apply Equation 7 to Equation 8, we have a new  equation:

i_s = v_s(1/R₁ + 1/R₂ + 1/R₃) (9)

from which the equivalent resistance is,

i_s/v_s = 1/R_eq = 1/R₁ + 1/R₂ + 1/R₃ (10)

A quick tip to find the equivalent resistance when we are dealing with only two resistors connected in parallel: the equivalent resistance can be obtained by dividing the product of the resistances by their sum. It is important to note that this formula is applicable exclusively to the specific scenario of two resistors in parallel (Riedel & Nilsson, 2015, 60).

Figure 3

From Figure 3, the equivalent resistance is,

R_{eq =} R₁R_2/R_1 + R₂ (11)

Voltage Divider

A voltage divider is a circuit that divides the input voltage into smaller, adjustable voltages. It consists of resistors connected in series. By varying the resistance values, we can control the output voltage across specific resistors, enabling us to power different components with varying voltage requirements.

Figure 4

We use Kirchhoff's voltage law (KVL) and Kirchhoff's current law (KCL); a blog about these two can be found in "What Is Voltage Law" to derive the voltage (v₁ and v₂)  from Figure 4 (Riedel & Nilsson, 2015, 61).

v_s= iR1 + iR2 (12)

i = v_s/ R_1 + R₂ (13)

Apply Equation 13 to the voltage equation  v₁ and v₂ :

v₁ = iR1 =  v_s (R1/R_1 + R₂)(14)

v₂ = iR2 = v_s (R2/R_1 + R₂)(15)

Current Divider

Similar to voltage dividers, current dividers allow the division of current among different paths. By using resistors in parallel, we can distribute the total current into smaller currents that flow through individual branches of the circuit. This technique finds applications in situations where precise current allocation is needed.

Figure 5

From Equation 11, we can obtain the voltage (v) from Figure 5.

v = ( R₁R_2/R_1 + R₂) i_s (16)

From Ohm's law, we know the voltage across the two resistors are,

v = i₁R₁ = i₂R₂ (17)

Replacing Equation 16 into Equation 17, we have

i₁ = ( R_2/R_1 + R₂) i_s (18)

i₂ = ( R_1/R_1 + R₂) i_s (19)

Equation 18 and Equation 19 demonstrate that when two resistors are connected in parallel, the current splits in such a way that the current flowing through one resistor is equal to the total current entering the parallel combination, multiplied by the resistance of the other resistor, and divided by the sum of the resistances (Riedel & Nilsson, 2015, 63).

Measure Voltage and Current

To understand and analyze electrical circuits, it is essential to measure voltage and current accurately. Voltage can be measured using a voltmeter, a device connected in parallel across a component to measure the potential difference. Current, on the other hand, is measured using an ammeter, which is inserted in series with the component to measure the flow of electrons (Riedel & Nilsson, 2015, 66).

Now, we have a multimeter (Figure 6) which can measure both the voltage, current, and other aspects. You can check out this website on how to utilize the multimeter properly.

Figure 6

Conclusion

Electrical resistance, a concept rooted in the works of Georg Simon Ohm, plays a vital role in understanding and manipulating electrical circuits. From resistors in series and parallel to voltage dividers and current dividers, each concept opens a realm of possibilities in electrical engineering. By effectively measuring voltage and current and employing delta to wye conversions, engineers can design and analyze complex electrical networks. Embrace the power of electrical resistance and unlock the true potential of modern technology.

Amplify your electrical engineering exam prep with School of PE. Our comprehensive courses come with what you need to succeed on exam day! Check out our FE Electrical or PE Electrical exam review courses now.

References

Riedel, S. A., & Nilsson, J. W. (2015). Electric Circuits. Pearson.

About the Author: Khoa Tran

Khoa Tran is an electrical engineer working at the Los Angeles Department of Water and Power and is currently pursuing his master's in electrical Power from the University of Southern California. He is fluent in both Vietnamese and English and is interested in outdoor activities and exploring new things.

Sparking Curiosity: What is Electric Charge?

The modern world runs on electricity; every day, we use it to power our homes, charge our devices, and light up our streets, but have you ever wondered what exactly electricity is? Simply put, electricity is the phenomenon resulting from the movement of an electric charge. Together in this blog, we will explore the history of electric charge's discovery, what it is, and the fundamental principles that govern it.

In this Blog,

1. History

2. What is Electric Charge?

3. Principle of Conversion

4. Conclusion

History

The very first instance of electric charge can be traced back to the ancient Greeks. They first noted that rubbing certain materials together, such as fur and amber, would cause them to attract or repel one another. Later, in the 1700s, American scientist Benjamin Franklin conducted his own series of experiments that led him to coin the usage of positive and negative charges. He added that electricity was not generated but transferred from one material to the other (Freedman et al., 2012, 688).

In 1897, British physicist J.J. Thompson, through his experiments using cathode ray tubes, discovered the electron. A cathode ray tube (CRT) is an electronic device that was once widely used in television sets, computer monitors, and other outdated electronic devices. Inside these CRTs was an electron gun that produced a beam of electrons accelerated by an electric field toward a fluorescent screen (University of Oxford, n.d.). The discovery of the electron revolutionized the field of physics; it explained how electricity is generated and established the foundation for the development of future electronics.

What Is Electric Charge?

Electric charge is an intrinsic property of matter, which serves as a fundamental attribute that defines the interaction and behavior of objects in the presence of electric fields. Electric charge quantifies the amount of electrical energy an object holds, playing a crucial role in various aspects of our lives. The unit of measurement of an electric charge is Coulomb (C), named after French physicist Charles-Augustin de Coulomb.

From his own experiment, Benjamin Franklin observed that objects that had gained electrons were negatively charged, while objects that had lost electrons had a positive charge. Electric charges, whether positive or negative, can interact with one another. Much like a magnet, when two charges of the same polarity - either positive or negative - are brought near one another, they will repel each other. However, when two charges of opposite types are brought near each other, they will attract each other and try to come together (Freedman et al., 2012, 688).

Electric charge can be visualized as a property that exists on the surface of an object. This property can be either positive or negative. The charge is distributed across the surface of the object and can be calculated using the following equation (Boston University, 1999):

Q = ne (1)

Where Q is the electric charge, n is the number of electrons, and e is the charge of a single electron.

Principle of Conservation

There are two fundamental universal principles that govern electric charge: the principle of conservation of energy and the principle of conservation of charge.

1. Principle of Conservation of Energy

The principle of conservation of energy states that energy can neither be created nor destroyed, but it can be converted from one form to another (University of Calgary, n.d.).

Using a simple closed-loop circuit, a battery connected to a lightbulb, we can visualize the conservation of energy. The chemical energy stored in the battery is then converted into electrical energy, which flows through the circuit and is converted into light and heat energy by the light bulb. Throughout the entire process, the total energy of the system remained constant. Thus, no energy was created nor destroyed; rather, it was transformed from one form to another.

2. Principle of Conservation of Charge

The principle of conservation of charge states that the total amount of electric charge in a closed system is constant (Freedman et al., 2012, 690).

When two neutrally charged objects, such as a plastic rod and a piece of fur, are rubbed together, they can become charged. In this instance, the rod gains a negative charge while the fur gains an equal amount of positive charge. This means that the total amount of electric charge in the system remains constant. The charge is transferred from one body to another and not created or destroyed. This principle of conservation of charge is believed to be a fundamental law of nature, and it has never been observed to be violated. Even in high-energy interactions where particles are created and destroyed, such as the creation of electron-positron pairs, the total charge of a closed system remains constant.

To illustrate both the principle of conservation of energy and conservation of charge, we can consider the example of a battery connected to a light bulb. When the circuit is closed, a flow of electrons occurs in the wire connecting the battery to the bulb, causing the bulb to light up. During this process, the battery converts chemical energy into electrical energy, which is transferred to the bulb as an electric charge. However, the total amount of charge in the circuit remains constant, meaning that the amount of charge leaving the battery is equal to the amount of charge arriving at the bulb. When the circuit is opened, the flow of electrons stops, and the energy is stored in the electric field created by the separation of charges in the wire and battery.

Conclusion

Electric charge is a fundamental property of matter that has revolutionized our understanding of electricity and electronics. The principle of conservation of electric charge, which states that the total amount of charge in an isolated system remains constant, is closely related to the principle of conservation of energy, and both principles play a crucial role in our understanding of the behavior of electricity. These principles have enabled us to develop devices such as batteries, light bulbs, and transistors that have transformed our lives.

Ready to supercharge your studies in electrical engineering? Check out School of PE's FE Electrical and PE Electrical exam prep courses now! We look forward to supporting your success.

References

Boston University. (1999, July 6). Electric charge and Coulomb's law. Physics. Retrieved May 14, 2023, from http://physics.bu.edu/~duffy/py106/Charge.html

Freedman, R. A., Ford, A. L., & Young, H. D. (2012). Sears and Zemansky's University Physics: With Modern Physics (A. L. Ford, Ed.). Addison-Wesley.

University of Calgary. (n.d.). Law of conservation of energy. Energy Education. Retrieved May 14, 2023, from https://energyeducation.ca/encyclopedia/Law_of_conservation_of_energy

University of Oxford. (n.d.). Cathode ray tube. Oxford Department of Physics. Retrieved May 14, 2023, from https://www2.physics.ox.ac.uk/accelerate/resources/demonstrations/cathode-ray-tube

About the Author: Khoa Tran

Khoa Tran is an electrical engineer working at the Los Angeles Department of Water and Power and is currently pursuing his master's in electrical Power from the University of Southern California. He is fluent in both Vietnamese and English and is interested in outdoor activities and exploring new things.

The Power of Networking: Expanding Opportunities for Your Team

Career success depends on networking opportunities. In fact, it is such a vital facet of career progression that most jobs obtained aren't even facilitated by online job boards and are instead found and offered at, or because of, in-person events where people can interact and gain insights into the person they'll potentially be working with. That's not to say that online networking can't lead to job opportunities.

In fact, regular online networking can lead to long-term job opportunities as well. In Zippia's survey on the power of making connections in the workplace, 78% of startups owe their success to networking, and employee referrals account for 40% of all hires. It goes so far as to state that 70% of people got their current job through networking. That's 70 people out of 100 who went to an event or held a casual conversation that led to a job opportunity for them.

This blog will take a look at the benefits of networking, powerful networking opportunities for your team, and the different places you can look to find these opportunities.

In this blog,

1. Using Networking Opportunities to Your Advantage

2. Powerful Network Expanding Opportunities for Your Team

3. Types of Networking

Using Networking Opportunities to Your Advantage

The benefits of networking for your team are vast.

• Build Your Reputation. Provided you've laid the groundwork for a highly proficient and productive work culture, engaging in networking opportunities can help the team build a positive reputation among clients, peers, and others they'd like to work with.
• Showcase Your Strengths. You have a team of excellent workers who do well with each other and people outside of their wheelhouse. They work well together. They have well-developed communication skills. They have a proven track record of completing jobs thoroughly and consistently. These are all qualities that prospective clients and other professionals are interested in knowing. Networking in various environments allows your team to showcase these quantifiable skills
• Create Awareness and Gain Visibility. Perhaps the biggest purpose behind networking is to create awareness of your brand and gain visibility within your industry.
• Gather Industry Insights. Another facet of networking that can benefit the team now and in the future is to gain industry insights. Learn about growing trends and find marginalized groups that haven't been tapped into for business relationships and opportunities. Learn what's working from people who've tried and failed to do what you're attempting before you attempt it.
• Gain Better Interpersonal Skills. Learning how to communicate with new people on a regular basis can help a person grow in confidence, as well as useful skills such as listening for the sake of understanding a problem rather than finding a band-aid solution. Learning to relate to people at a personal level can take your team far.
• Get Advice from Seasoned Professionals. Whether youre new or a seasoned professional, you're always going to find somebody more skilled or experienced in an area than you are. Humbling yourself enough to listen to advice and cautionary tales from people who've done it is a valuable skill. It may not discourage you from following the same course of action, but it will give you the context of the task within the industry.
• Develop Long-Lasting Relationships. The people you meet aren't solely for one event or one task. Networking among colleagues and industry professionals can help develop lifelong relationships that help with the long-term success of a person's career. Of course, some interactions can end within a year or two, but the great ones will last the length of a person's lifetime and become a valuable tool for your team.
• Develop Useful Soft Skills. Soft skills, as opposed to hard skills that are quantifiable and produce product results within a company, are more personality traits, like leadership and communication, negotiation, etc. These skills will follow a person their entire life, meaning their entire time within your team. Offering them the opportunity to gain these skills can help them manage stressful and unforeseen circumstances.

Powerful Network Expanding Opportunities for Your Team

One question you may ask is, "Where do I go to give my team these opportunities?" There are so many different places to go that you can utilize to your advantage, and some of them may surprise you.

1. Industry-Specific Speaking Engagements. Conferences are a bigger deal for professionals than ComiCon makes it seem. Not only will you find information from various levels within your industry, but you'll also find a jumping-off point for people you may want to work with in the future. For instance, you have an idea for a project you'd like to work on. Attending conferences and panel events gives you a starting point for communication. Slightly less cold than a cold introduction, an email or phone call can begin with, "Hi, I loved your lecture at (insert conference here), and I'd love to ask you a few questions." From there, you'll gain a new contact, with the added benefit that they will know who you are. Soon, you'll be able to discuss your project and make possible offers for working together.
2. College and University Lectures. Lectures can provide you with a professional whose job is connecting people. Professors are there for educational purposes and provide a place for people to get to know each other in a comfortable place; a place that allows them to practice their communication skills and find others whose goals align with their own. Don't discount schools as being a networking tool. Some teams that started in college twenty years ago are still together today.
3. Interviews. Interviews can sometimes seem like they are very one-sided and not for your personal use, but similarly to conferences, they play a powerful role in networking with the added benefit of a personal touch. Conferences are there for a larger audience. That larger audience gets boiled down to you. In contrast, interviews are far more personal. They begin with you and expand to a wider audience. You have the opportunity to add your personal touch to the interaction, no matter what the context.
4. After Hours Casual Meetings. This one is pretty simple. You attended a panel event, which in itself is an excellent networking opportunity. You've met with industry professionals and listened to others talk for hours. You encounter a group of people that you clicked with, and they invite you for drinks or dinner at a local restaurant. This is an excellent form of relationship building and a powerful opportunity for you to expand your reach and build a network of trustworthy, knowledgeable, and dependable people in your industry.

Types of Networking

It is astounding to think about the way that networking has evolved in the last century. Word of mouth is still a big part of gaining new opportunities, but we have the ability to put ourselves into the public eye like never before. For this reason, social media is at the top of this list.

• Social Media Events: Social media offers a greater opportunity to balance the benefits of several types of networking. Its accessibility allows attendees to be present from hundreds of miles away, and due to the difficulties of maintaining conversation with a wider audience, often, the events are smaller and allow for more personal connection than larger conferences.
• Casual Networks: Networking through family and friends is a good way to get your feet wet. Close relationships are a valuable asset in expanding your reach, whether you're a freelancer or the CEO of a Fortune 500 company. Utilizing the people you know to ask questions or get in touch with somebody who has a specific skill set is a valuable opportunity in itself.
• Professional Associations: Industry-specific clubs like writers' guilds, the American Nurses Association, construction associations, and many others provide a space to find individuals specific to your needs. You can connect with people who understand industry standards and have a proven track record in your field. Using these associations is an easy path to networking because oftentimes, they'll have a directory of people who are already okay with being contacted for specific services and information
• Community Service Clubs: Don't underestimate area-specific community clubs. They provide an excellent opportunity to build friendships with people you may not have met outside of the club. It's a low-key way of finding people who may (or may not) have specific information geared toward your industry, but you'll find valuable skills in other areas, and those relationships can always lead to more.

The opportunities that networking can give your team to expand their reach and create lasting business and personal relationships will affect how their job is done and how the team is seen from an outside perspective. Specifically, sourcing information and gaining insights into industry standards via networking opportunities enables your team to build their reputation, showcase your strengths, and create awareness of the overall efficiency and culture behind your team's success.

Are you interested in professional development and learning opportunities for yourself, your academic institution, or your business? School of PE can help! Get in touch with us today to learn more.

About the Author: Anna Taylor

Anna Taylor is a freelance writer and avid researcher- a jack of all trades, but a master of none. She graduated from the University of Hawai'i with an Associates Degree in Liberal Arts because she had no idea what she wanted to be when she grew up. She has since found her love of Extended Reality and the possibilities it brings to the world, as well as gardening, cooking, and writing. Anna lives in Interior Alaska with her family.

Exploring Electromagnetic Inductance

Index:

1. History

2. What is an Inductor?

3. Inductance Connection

4. Types of Inductors

5. Application

6. Conclusion

History

In 1831, English natural philosopher Michael Faraday discovered electromagnetic inductance. From his discovery, he noted that when a current flows through a conductor, a magnetic field is produced around it. This resulting magnetic field also induces an electromagnetic force (EMF) in the same conductor, which opposes the current flow. This phenomenon is called self-induction or simply inductance.

Despite being originally discovered by Faraday, Joseph Henry, an American scientist, was credited for making significant contributions to the development of electromagnetism, leading to the unit of measurement for an inductor to be named after Henry. Working independently of Michael Faraday, Henry demonstrated that an electric current could be induced in a coil of wire by a changing magnetic field, setting up the groundwork and foundation for future developments of inductors and transformers (Whelan et al., n.d.). The unit "Henry" was first proposed in 1893 by the International Electrical Congress and was later adopted as the standard unit of inductance by the International System of Units (SI) in 1946.

What Is an Inductor?

An inductor is a passive element, which stores energy in its magnetic field when energized (Figure 1). It is typically made with coiled wire wrapped around a conductive metal core. An inductor opposes the changes in the current passing through it. The shape of the core can vary depending on the type of inductor, but the most common shapes are cylindrical, toroidal, and rectangular.

Figure 1

Inductance is a circuit parameter that describes an inductor and is measured in henrys (H), and the letter L represents inductance. The passive sign convention is used to assign the current reference direction in the direction of the voltage drop across the inductor, as shown in Figure 2.

Equation 1 reflects this convention, and if the current reference is in the direction of the voltage rise, it is written with a minus sign.

v = L di/dt (1)

The voltage across an inductor's terminals is proportional to the rate of change of the current flowing through the inductor, as shown in Equation 1. From this equation two observations can be made from this relationship.

If the current is constant, then the voltage across an ideal inductor is zero, making it behave as a short circuit in the presence of a constant or DC current.

If the current cannot change instantaneously in an inductor, then it is impossible to change it by a finite amount in zero time.

From the above observations, an infinite voltage will theoretically be generated if infinite self-inductance occurs, which is physically impossible (Nilsson & Riedel, 2015, 176).

We can rearrange Equation 1 to obtain the current equation.

i(t) = 1/L ∫₀^t vdx + i(t₀) (2)

The inductor energy equation can be expressed as:

w = 1/2 Li²       (3)

Inductance Connection

For the simplicity

1. Series

In a series connection, inductors are connected from end-to-end (Figure 3) so that the current flows through each inductor remain the same, similar to resistors being connected in series. The inductors can be simplified to a single equivalent inductor. It can be achieved by summing the individual inductances (Nilsson & Riedel, 2015, 187).

Figure 3

The equivalent inductance equation can be expressed as:

L_eq = L₁ + L₂ + ... + L_n (4)

The voltage across the terminal can be found by summing the voltage of each inductor:

v = v_L1 + v_L2 +...v_Ln  (5)

2. Parallel

In a parallel connection, the inductors are connected side-by-side (Figure 4) and can be simplified into an equivalent circuit by summing the reciprocals of each inductor's inductance.

Figure 4

The equivalent inductance can be expressed as:

 1/L_eq = 1/_L1 + 1/_L2 + ... + 1/_Ln  (6)

From Figure 3, we can observe the terminal current  equals the sum of the other inductor currents.

 i = i_L1 + i_L2 + ... + i_Ln  (7)

Types of Inductors

Here, we will introduce three of the most common types of inductors in use: air-core inductors, iron-core inductors, and toroidal inductors.

1. Air-core Inductor: Air-core inductors are different from the other two inductor types because they are made with a coil of wire wrapped around a non-magnetic core, like plastic or ceramic. This type of inductor is used in high-frequency applications, where its low inductance and low loss make it an ideal choice. These are often used in radio tuning circuits.

2. Iron-core Inductor: Iron-core inductors are made with a coil of wire wrapped around a ferromagnetic core, like iron or ferrite. With a higher inductance and high saturation flux density, this inductor type is commonly used in low-frequency applications. These are often used in power supply and audio circuits.

3. Toroidal Inductor: Toroidal inductors are made with a coil of wire wrapped around a toroidal (ring) core; this core is typically made of iron or ferrite. This shape allows for a lighter-weight design and higher inductance. This inductor type is commonly found in noise filtering and power supplies.

Application

Thanks to inductors, engineers have been able to utilize its energy storage in multiple real-world applications.

1. Filter

An inductor's ability to prevent instant current changes ensures a constant voltage output. A filter circuit consists of an inductor and a capacitor to smooth out the DC voltage, ensuring a stable and regulated power supply.

Not only are they used to filter noise from the current of a power circuit, but inductors are also used to filter out unwanted signals and noise. A choke coil, which is a type of inductor, is used to block high-frequency noise and prevent it from entering the audio amplifier.

2. Transformer

A transformer is a device that transfers electrical energy from one circuit to another through the properties of electromagnetic induction. A transformer consists of two or more coils of wire wrapped around a ferromagnetic core. Transformers are used to step up or step-down voltage levels in AC power supply circuits, making them essential for the transmission and distribution of electrical power.

 

Conclusion

Inductors are electronic components that store electric energy through their magnetic fields. Through them, we can filter out unwanted noises in power and signals and transfer and manage electrical energy across a circuit. They are essential for the proper functioning of power supply circuits, transformers, audio circuits, radio circuits, and oscillators, among other applications.

Looking to electrify your electric engineering exam prep? Don't miss School of PE's FE and PE Electrical exam prep courses. Register now on www.schoolofpe.com.

References

Nilsson, J. W., & Riedel, S. A. (2015). Electric Circuits. Pearson.

Whelan, M., Reilly, E., & Rockwell, S. (n.d.). Joseph Henry - Engineering Hall of Fame. Edison Tech Center. Retrieved May 9, 2023, from https://edisontechcenter.org/JosephHenry.html

About the Author: Khoa Tran

Khoa Tran is an electrical engineer working at the Los Angeles Department of Water and Power and is currently pursuing his master's in electrical Power from the University of Southern California. He is fluent in both Vietnamese and English and is interested in outdoor activities and exploring new things.